Non-Equilibrium Gas Flow

The accurate modeling and simulation of non-equilibrium processes in rarefied gases or gas micro-flows is one of the main challenges in present gas dynamics. The traditional equations are known to loose their validity in extreme physical situations which exhibit strong non-equilibrium. In these classical models the non-equilibrium variables, stress tensor and heat flux, are coupled to gradients of velocity and temperature as given in the constitutive relations of Navier-Stokes and Fourier (NSF). These relations are valid close to equilibrium, however, in rarefied gases or micro-flows the particle collisions are insufficient to maintain equilibrium. Away from equilibrium higher order multi-scale relaxation processes in the fluid become dominant and essential.

Modeling in Kinetic Gas Theory

Kinetic gas theory is based on a statistical description of the gas and provides a valid framework to describe processes in a rarefied regime or at small scales. The main variable used to describe the gas is the distribution function or probability density of the microscopic particle velocities which describes the number density of particles with certain velocity in each space point at a certain time. The evolution of the distribution function is given by the Boltzmann equation, a 7-dimensional integro-differential equation. However, in many situations this detailed statistical approach yields a far too complex description of the gas. Instead, it is desirable to have a continuum macroscopic model based on partial differential equations for the fluid field variables. This model should accurately approximate the multi-scale phenomena present in kinetic gas theory in a stable and compact system of field equations.

The main scaling parameter in non-equilibrium gas flows is the Knudsen number Kn. It is computed from the ratio of the mean free path between collisions of gas particles and a macroscopic length. Perfect equilibrium is given in the limit Kn=0, described by the dissipationless Euler equations of gas dynamics. The system of NSF is capable to compute some non-equilibrium effects, but in many processes failures occur at about Kn=0.01. For large Knudsen numbers the collisions between the particles can be neglected and they move ballistically in a free flight. Between these limiting cases lie the transition and kinetic regime. In the kinetic regime the non-equilibrium is so strong that a detailed description by a distribution function becomes necessary, either by using the Boltzmann equation or the direct simulation Monte-Carlo (DSMC) method. However, in the transition regime a fluid description is still possible, although a larger set of field variables and/or higher derivatives may be needed. In this regime Boltzmann simulations or DSMC become increasingly expensive, favoring the use of continuum models.

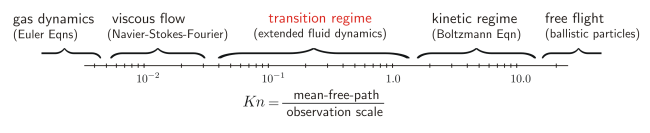
The limit for quantitative predictive solutions of advanced continuum models is currently around Kn=1, depending on the process. Qualitative behaviour can be predicted at larger values of Kn. One aim of the research at ACoM is to establish continuum models as a useful tool to compute non-equilibrium gas flows and to push their limit further.

Regularized Moment Equations

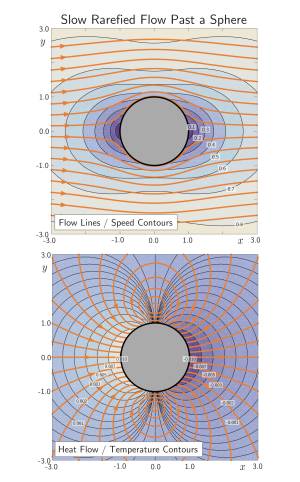
In moment approximations the distribution function is replaced by a finite vector of fields in every space point, which represent moments of the distribution function. The first moments turn out to be the fluid dynamics fields of density, velocity and temperature. Higher moments include the stress tensor and heat flux. As such the usage of moments is inherently linked to a physical description. Mathematically, moment approximations represent a complexity reduction from an infinite dimensional phase space to a finite dimensional space of field variables. Slow Rarefied Flow Past a Sphere: Stream lines and heat flow lines The actual derivation of moment equations combines elements from numerical approximation techniques, asymptotic expansions and PDE theory. The use of moment equations in kinetic gas theory has been pioneered by Harold Grad in the 50s of the 20th century. They have been developed as alternative to the Burnett equations which have been derived formally by a Chapman-Enskog expansion of the Boltzmann equation. However, both approaches suffered from artefacts and mathematical instabilities, as well as inaccurate results. Recently, a regularization technique has been developed which combines the Chapman-Enskog expansion with the moment approximation and yields stable and accurate field equations.

One result of the new theory are the Regularized 13-Moment-Equations (R13). They describe the rarefied or micro-flow of a gas by 13 field quantities, that is, the density, velocity, temperature, pressure deviator (trace-free stress tensor) and the heat flux. Each quantity follows its own evolution equation and the whole set forms a system of parabolic-hyperbolic partial differential equations with relaxation. In contrast to Grad's moment equation the R13 theory adds dissipative gradient expressions to the first order hyperbolic flux function, which parabolize the system. These diffusive parts represent higher order kinetic dissipation processes relevant to rarefied gas flows. After developing the theory of regularized equations also wall boundary conditions could be derived based on the boundary conditions available in kinetic gas theory. The mathematical structure of the R13 equations resembles that of classical fluid mechanics. They can be written in balance law form and are accessible by standard numerical methods like Finite-Volume or Finite-Element approaches. In simplified geometries for particular flow situations, they allow also to find analytical solutions. These solutions provide additional intrigueing insight into the peculiarities of rarefied flows which often show anti-intuitive behaviour. The figure on the right shows the slow flow past a sphere in a rarefied situation together with the heat flux pattern. Note, that the heat flux flows from hot to cold regions in contrast to Fourier's law.

For an introduction into the engineering aspects of the Regularized 13-Moment-Equations, have a look at the Lecture Notes (pdf, 2.6MB) of the Von-Karman Institute Lecture Series RTO194 (2011) on “Models and Computational Methods for Rarefield Flows”.